Quantum-well states at the surface of a heavy-fermion superconductor

Heavy fermions form a unique class of quantum materials that exhibit exceptional properties related to their narrow electronic-band dispersion¹²。Previous experiments on heavy fermions showed that reducing the dimensionality leads to enhanced electronic correlations and strong coupling superconductivity^15,16。Furthermore, narrow surface bands with a Dirac dispersion were found in a semiconducting heavy fermion¹⁷。In spite of intensive investigations, 2DHFs have not been observed at surfaces of superconducting compounds and no quantum-well states owing to lateral confinement of heavy electron states have been realized.

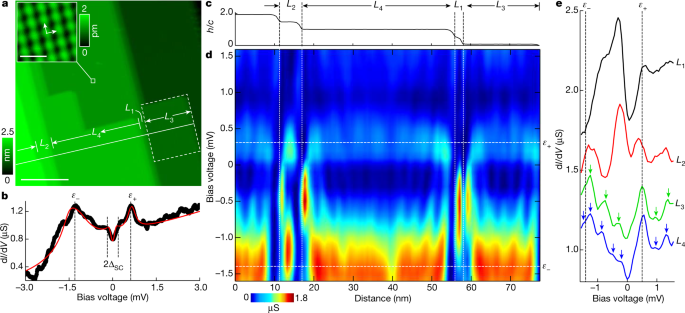
We investigate the heavy-fermion superconductor URu₂Si₂, which exhibits correlated narrow electron bands crossing the Fermi level and undergoes a transition to the HO phase characterized by an as yet unknown order parameter¹⁴。A partial gap opens in the electronic band structure belowT_HO = 17.5 K, out of which an unconventional superconducting state develops belowT_c = 1.5 K (refs. ^14,18）.The surface electronic states observed until now mostly have small effective masses^19,20,21,22。Using STM to investigate small-sized, atomically flat terraces on the U-terminated surface of URu₂Si₂at sub-kelvin temperatures, we observe clearly 2DHFs with an effective mass 17 times the free electron mass, as well as quantization owing to lateral confinement and uncover the surface–bulk interaction. In Fig.1a, we present a STM image of terraces of U-terminated surfaces on URu₂Si₂(see Extended Data Fig.1for the surface termination). Our starting point is the tunnelling conductance obtained in a small range of a few mV around the Fermi level and at a temperature of 0.1 K, well below the superconducting critical temperature, shown in Fig.1b(see Extended Data Fig.2for a larger bias voltage range).

We focus on the tunnelling conductance along the white line shown in Fig.1a。Along this line, we identify four terraces of different sizes,L₁ ≈ 2 nm,L₂ ≈ 5.5 nm,L₃ ≈ 20 nm andL₄ ≈ 38.5 nm (Fig.1c）.We observe a strong bias voltage and position dependence of the tunnelling conductance, which is different for each terrace as illustrated in Fig.1d。We show in Fig.1erepresentative tunnelling conductance curves at each terrace, in which we identify a set of regular peaks.

Let us analyse the terraceL₃(dashed rectangle in Fig.1a）.We present a symmetrized map of the tunnelling conductance in Fig.2a(seeMethodsfor further details). We identify a set of peaks in the tunnelling conductance, which evolve in both position and bias voltage. Subtracting the features atε₋andε₊(details provided inMethodsand in Extended Data Figs.3and4), we obtain the pattern shown in Fig.2b, which shows the lateral quantization of 2DHFs. The quantization pattern for confined electrons resembles the Fabry–Pérot expression for an interferometer made by partially reflecting mirrors²³(reflection coefficientrand the phase shiftϕare the free parameters; details in Extended Data Fig.4and results on different terraces in Extended Data Fig.5）.Lateral quantization results from interfering wavefunctions partially reflected at steps. Quantized levels obtained from the Fabry–Pérot expression are the white dots in Fig.2a,b, whose position coincides well with the peaks in the conductance pattern observed in the experiment. In Fig.2c, we plot as points the position of the peaks as a function of the wavevectorkand as a line the dispersion relationE = E₀ + ħ²k²/ (2m*), in whichm* is the effective mass andE₀the bottom of the band. We obtainE₀ = −2.3 meV andm* = 17m₀, withm₀the free electron mass, that is, we find that the 2DHF is derived from a massive surface electron state. A detailed comparison of the tunnelling conductance versus position with the square of wavefunctions confined by a lateral potential leads to excellent fits, shown in Fig.2d。The phase shiftϕdetermines the position and energy of the peaks (white dots in Fig.2a,b) and the best account of our observations is obtained withϕ = −π. We find values aroundr ≈ 0.2, which slightly increase when approachingE₀(Fig.2e）.The low value ofris also found in surface states of simple metals; for example,ris between 0.2 and 0.4 in Ag and Cu (refs. ^23,24,25）.However, the energy dependence (Fig.2e) at the surface of URu₂Si₂is completely different to that in usual metals. Althoughrvaries mildly in Cu or Ag in the range of a few eV (see dashed line in Fig.2eand refs. ^23,24,25), here we observe instead thatrdecreases markedly in a range of a few meV (points in Fig.2e）.We can reproduce the observed dependence ofrversus bias voltage assuming a potential well^23,24(continuous line in Fig.2e; details provided inMethodsand Extended Data Figs.3and4）.It is also insightful to trace the tunnelling conductance as a function of the bias voltage at the centre of the terrace in Fig.2b(circles in Fig.2f) and compare it to the expectation forr ≈ 1 (dashed line in Fig.2f) and forr ≈ 0.4 (continuous line in Fig.2f）.We see that, for a reducedr, both the periodicity and shape of the tunnelling conductance are well explained in an energy range of a few meV, that is, two orders of magnitude below the energy range observed in conventional metals^{1,2,3,4,23,24,25,26}。

To further investigate the quantized levels, we have fitted each peak to a Lorentzian function, whose width Γ (black arrow in Fig.2b) provides the lifetimeτ(Fig.2g) of the quantum-well states. Taking a two-dimensional electron gas, we expect\(\hbar /\tau ={\Gamma }_{0}+(| {E}_{0}| /4\pi ){\left[(E+| {E}_{0}| )/{E}_{0}\right]}^{2}\)\(| {\rm{ln}}| (E+| {E}_{0}| )/{E}_{0}| -{\rm{ln}}(2{q}_{{\rm{TF}}}/{k}_{{\rm{F}}})-1/2| \), withħthe reduced Planck’s constant,q_TF = 0.0906 Å⁻¹the Thomas–Fermi screening length andk_Fthe Fermi wavevector²⁷(dashed line in Fig.2g）.Our data are not well reproduced by this expression. Taking instead\(\hbar /\tau ={\Gamma }_{0}+(| {E}_{0}| /4\pi ){\left[(E+| {E}_{0}| )/{E}_{0}\right]}^{2}\)withE₀ = −2.3 meV and Γ₀ ≈ 60 μeV (continuous line in Fig.2g), we find a much better account of our data^27,28。The latter expression takes into account the connection between the 2DHF and bulk states, showing that, in our experiments, the lifetimeτis set by the decay of the 2DHF into heavy-fermion bulk states. Quantum-well states sense the bulk correlations, given by the quadratic energy term inħ/τ。This has been observed in surface states of noble metals, monolayers of Pb and in Sb. However, in those cases, the energy range was three orders of magnitude above the one we discuss here^3,6,11,28。

From the obtained value of Γ₀ ≈ 60 μeV, we estimate the lifetime of the ground state asτ₀(E₀ = −2.3 meV) = ħ/Γ₀ ≈ 11 ps. Similarly, the lifetime of states close to the Fermi level isτ(E = 0) = ħ/Γ(E = 0) ≈ 3 ps. We can also estimate a value for a mean free path,ℓ₀ = v_Fħ/Γ(0) ≈ 0.14 μm, withv_Fthe Fermi velocity of URu₂Si₂。This value is on the same order of magnitude as those observed in ultraclean URu₂Si₂single crystals²⁹。

To vindicate the existence of a heavy-fermion surface state, we performed density functional theory calculations of the surface band structure of a slab of URu₂Si₂(Extended Data Fig.6）.We find a shallow, U-derived f-electron band with a flat dispersion relation compatible with our experiments around theXpoint of the simple tetragonal Brillouin zone. The bulk electronic spectrum is gapped in this part of the Brillouin zone^21,22。The rest of the Brillouin zone provides surface states with much smaller effective masses.

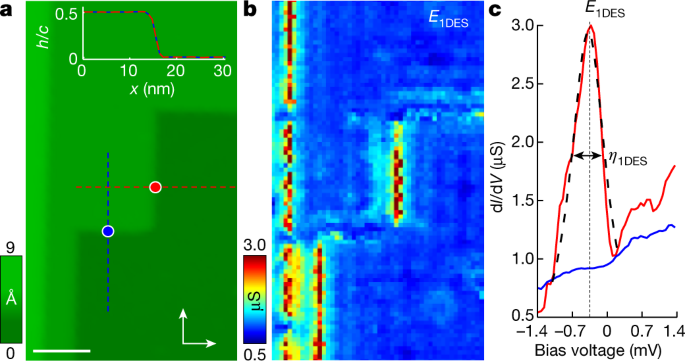
In Fig.3, we show that the 2DHF is peculiarly modified at the steps separating terraces at which one-dimensional edge states (1DESs) appear. 1DESs were previously observed in simple metals, in which a gap opens at the step and is filled with a very large density of states atE_1DESby the 1DES^{3,8,11,24,25,30}。The width of the conductance peak atE_1DES,η_1DES, results from inelastic scattering into bulk states^24,25。Here we find that the features in the tunnelling conductance completely change at a step (Fig.3a,b）.We find a high peak atE_1DES ≈ −0.38 meV (Fig.3b,c) in steps, but notably only along one of the two equivalent in-plane axes, that is, an in-plane symmetry breaking occurs. The HO is known to cause breaking of the body-centred translation symmetry¹⁴, leading to inequivalent electronic properties in subsequent U layers, which is consistent with our measurements (details inMethodsand Extended Data Fig.7）.This should reduce inelastic scattering and favour the formation of a 1DES. Here, however, we observe a rather unique situation in which the edge state is either observed or not, on two crystallographically equivalent in-plane axes. This indicates spontaneous symmetry breaking of the fourfold rotational symmetry close to the surface. Such in-plane symmetry breaking has been proposed³¹for bulk URu₂Si₂but has been difficult to detect. This symmetry breaking would cause an orthorhombicity given by a tiny difference in the basal-plane lattice constantsaandb(\(\frac{| \,a-b\,| }{(a+b)}\approx 1{0}^{-5}\))³²。Other experiments could, however, not confirm such in-plane symmetry breaking³³, which could be favoured by defects. Recent group theory considerations and nuclear magnetic resonance (NMR) data propose that the HO state can belong to four space groups, #126, #128, #134 and #136, all having the same crystal structure as the high-temperature state³⁴。NMR experiments suggest the presence of fourfold symmetry at Ru, Si and U sites, narrowing down the most probable choice to #126 (refs. ^34,35）.Notably, although these measurements gave absence of fourfold symmetry breaking in bulk URu₂Si₂,我们的STM数据敏感个体铀ers clearly show an in-plane symmetry breaking in the 1DES. We note that a possible source of changes in the electronic structure that could also affect the 1DES is modifications of the valence of U edge atoms²¹。尽管如此,对称性破1 des suggests a deeper origin, which pinpoints that the 1DES serves as a sensitive probe of fundamental electronic properties of the near-surface U lattice.

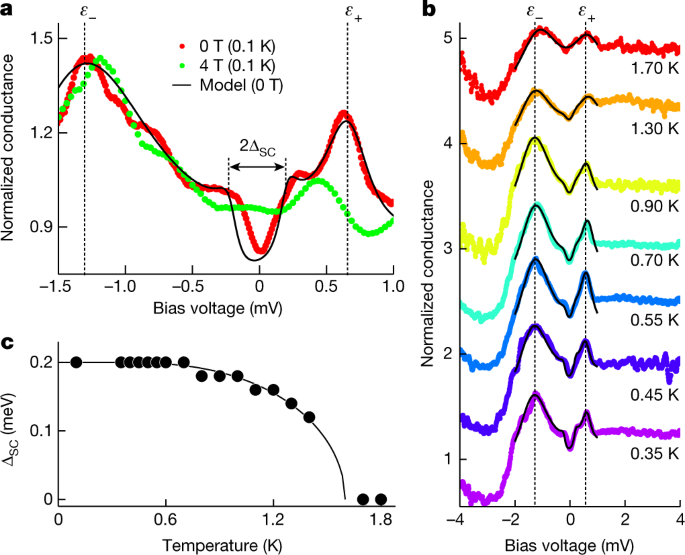
At the energy range below the superconducting gap, Δ_SC ≈ 200 μeV, we observe that there is a large zero-bias conductance (see Fig.4a）.There are indications for unconventional superconductivity in bulk URu₂Si₂, with a d-wave symmetry order parameter²⁹。This can contribute to the suppression of the superconducting features in the tunnelling conductance, but it hardly leads to the zero-bias conductance observed in our experiment. Similar small-sized superconducting features are found in other heavy-fermion superconductors, such as CeCoIn₅or UTe₂, and remain difficult to explain^36,37,38。Most notably, macroscopic measurements such as specific heat or thermal conductivity provide, in all these systems, a negligible zero-temperature extrapolation of the electronic density, suggesting that the superconducting density of states at the Fermi level is very small¹²。The 2DHF is strongly coupled to the superconducting bulk states and the proximity effect from bulk superconductivity should provide only a small amount of states at low energies. However, it is important to consider the coupling of the 2DHF to strongly energy-dependent resonant states giving peaks in the tunnelling conductance as well. The concomitant broadening then leads to a large zero-bias tunnelling conductance. Using a model that takes this into account (seeMethodsand Extended Data Fig.8), we can understand the main features of the tunnelling conductance and follow the superconducting gap with temperature (Fig.4b,c）.这解决了宏观之间的差异and surface experiments and shows the relevance of two-dimensional electronic states to understanding the tunnelling conductance.

In summary, we have observed 2DHFs in terraced surfaces inside the HO phase of URu₂Si₂。The 2DHF exhibits quantum-well states with energy separation of fractions of a meV when confined between steps. The 2DHF is connected to the bulk heavy-fermion states. At steps, we observe a 1DES, which shows in-plane electronic symmetry breaking and inequivalent electronic arrangement in subsequent U layers in the HO phase. The discovery of 2DHFs and related confined states opens new possibilities to study the interplay of quantized heavy-fermion states and unconventional superconductivity, as several heavy-fermion materials show unconventional superconductivity in the bulk, often coexisting with other long-range ordered phases. Apart from URu₂Si₂, there are other heavy fermions, such as CeCoIn₅, UBe₁₃, UPt₃or UTe₂, in which the proposed superconducting states are spin-singlet d-wave or spin-triplet p-wave and f-wave states. These could exhibit 2DHFs and the associated edge states could incorporate excitations with unique properties such as Majorana fermions following non-Abelian statistics. Furthermore, because the source of quantization is lateral confinement, correlated quantum-confined states can be obtained in nanostructures built on the surface by manipulation of adatoms or by controlling layer growth in thin films^15,16,39,40。This opens new avenues to generate, isolate and manipulate excitations in unconventional superconductors.

STM experiments

Single crystals of URu₂Si₂were grown by the Czochralski technique in a Tetra Arc Furnace. We scanned samples for a low residual resistivity and a high superconducting critical temperature, close to 1.5 K. Such samples were then cut in a bar shape with dimensions 4 × 1 × 1 mm³, with the long distance parallel to thecaxis. We mounted the samples on the sample holder of a scanning tunnelling microscope. The scanning tunnelling microscope was mounted in a dilution refrigerator. The resolution in energy of the setup was tested by measuring the superconducting tunnelling conductance with the tip and sample of s-wave superconductors Al and Pb down to 100 mK (ref. ⁴¹）.Details of image-rendering software are provided in refs. ^42,43。The scanning tunnelling microscope head features a low-temperature movable sample holder, which is used to cleave the sample at cryogenic temperatures^41,44。At the same time, and importantly for this study, the sample holder allows modifying many times the scanning window. The terraces discussed in this work were found in three different samples, after studying hundreds of fields of view.

Surface termination in URu₂Si₂

We focus on U-terminated surfaces. In Extended Data Fig.1a, we show the URu₂Si₂crystal unit cell highlighting the U, Si and Ru planes; their inter-layer distances are indicated in units of thec-axis lattice parameter. In Extended Data Fig.1b–d, we show STM images corresponding to different surface terminations. These surfaces are all obtained after cryogenic cleaving. On the surfaces full of square-shaped terraces, we find the results obtained in the main text. An example is shown in Extended Data Fig.1b。All the observed terraces are separated byc/2 ≈ 4.84 Å. In Extended Data Fig.1c,d, we show terraces with a triangular shape, in which we do not observe the phenomena discussed in the main text. Here the distance between consecutive terraces is about 0.11c, about 0.39cor about 0.61c, which correspond, respectively, to the three possible distances between U–Si planes (coloured arrows in Extended Data Fig.1a）.Therefore, we see that the surfaces with terraces having a triangular shape correspond to Si layers, sometimes with a U layer in between. By contrast, the surfaces with terraces having a square shape are U terminated. Atomically resolved images inside terraces (Extended Data Fig.1e) provide the square atomic U lattice with an in-plane constant lattice ofa = 4.12 Å . In Extended Data Fig.1f, we show a typical atomic-sized image on Si-terminated surfaces. We do not observe atomic resolution and have sometimes seen circular defects. Defects in the U-terminated surfaces are very different, as shown in Extended Data Fig.1g–o。We distinguish two distinct types of defect. The defects can be either point-like protrusions (Extended Data Fig.1g,h) or troughs (Extended Data Fig.1i）.Sometimes, defects are arranged in small-sized square or rectangular structures (Extended Data Fig.1j–o）.Most of these defects are probably because of vacancies or interstitial atoms in layers below the U surface layer.

Tunnelling conductance in the HO state

The tunnelling conductance of the HO state has been discussed in refs. ^{45,46,47,48,49}。We have reproduced the results, as shown in Extended Data Fig.2a,b。The tunnelling conductance results from simultaneous tunnelling into heavy and light bands, as in other heavy-fermion compounds^50,51,52。The red line in Extended Data Fig.2aforT = 18 K follows a Fano function

in whichAis a constant of proportionality,qis the ratio between two tunnelling paths andE_Fanois the Fano resonance energy with width\({\Gamma }_{{\rm{F}}}=2\sqrt{{(\pi {k}_{{\rm{B}}}T)}^{2}+2{({k}_{{\rm{B}}}{T}_{{\rm{K}}})}^{2}}\),T_Kbeing the Kondo temperature^45,46。For the fit, we include an asymmetric linear background owing to the degree of particle–hole asymmetry in the light conduction band^45,53。To account for the thermal broadening, we convolute the result with the derivative of the Fermi–Dirac distribution. We findq = 0.8 ± 0.5,E_Fano = 3 ± 1 mV, Γ_F = 22 ± 1 mV andT_K = 90 ± 5 K, consistent with previous reports^45,46。

Inside the HO phase (red line in Extended Data Fig.2b), we use the same Fano function, multiplied by an asymmetric BCS-like gap function with an offsetδ_E

The resulting function is convoluted with the derivative of the Fermi–Dirac distribution function. We findδ_E(4.1 K) = 1.5 ± 0.5 meV and Δ_HO(4.1 K) = 4.0 ± 0.5 meV, consistent with previous reports^45,46。

Note that we also observe further features at lower temperatures and smaller bias voltages (Extended Data Fig.2c）.The red line in Extended Data Fig.2cis a fit described below. The features above the superconducting gap can also be roughly obtained by using two Lorentzians atε₋andε₊and an asymmetric background. Probably, the peaks atε₋andε₊are because of avoided crossings in the band structure of the 2DHF at very low energies. We notice that the small feature atε₊occurs at a very similar energy range as a kink in the band structure found at the surface of Th-doped URu₂Si₂(refs. ^48,49）.In the calculations we show below, we can identify features in surface f-derived bands that can be associated to such peaks in the tunnelling conductance. However, such features can form as a result of correlations elsewhere in the Brillouin zone as well.

Quantum-well states at terraces between steps

In Extended Data Fig.3a, we show the tunnelling conductance background subtracted from Fig.2ato obtain Fig.2b。To carry out the background subtraction, we first identify the features atε₋andε₊in the conductance map. These are the light-blue regions centred atε₊and the red–yellow region centred atε₋in Extended Data Fig.3a。We then identify the edge states occurring at the steps, given by the red areas at the sides of Extended Data Fig.3a。Similar peaks are obtained on steps separating different terraces. The nature and shape of the 1DESs is discussed below. We then model these features by a set of Lorentzians and obtain the pattern shown in Extended Data Fig.3a。We subtract this pattern from the experiment (Fig.2a) to obtain the pattern shown in Extended Data Fig.3b。

To model the quantum-well states, we use the Fabry–Pérot interferometer expression for the density of statesg_FP(x, E) given by

with\(k=\sqrt{2{m}^{* }(E-{E}_{0})/{\hbar }^{2}}\),m* the electronic effective mass,rthe reflection amplitude,ϕthe phase andLthe width of the terrace²³。法布里-珀罗干涉仪是一种光学resonator made of semireflecting mirrors and provides a simple and insightful way to model electronic wavefunctions confined between two wells. More information on surface band structure and on quantum-well states by confinement is provided in refs. ^{3,9,54,55,56,57,58,59,60}。We assume a symmetric potential well withL = 20 nm,r = 0.5 andϕ = −π. The pattern generated by equation (3) is shown in Extended Data Fig.3c。White points provide the positions of quantized levels as in Fig.2a,b。It is not difficult to see that the structure of quantized levels is renormalized together with the electronic band structure. Smaller electronic effective masses imply larger quantized level width and separation, and vice versa. However, the simple Fabry–Pérot model does not take into account relaxation by electron–electron interactions, which lead to the extra level broadening discussed in the main text and in refs. ^27,28,61。

The black lines in Fig.2dare fits to the equation (3）.To account for the behaviour at the edges, we add the equation (5) for the 1DES. We use the parameters extracted for the terraceL₃, discussed in Extended Data Table1。We show further examples in Extended Data Fig.4。Note that, in Fig.2d, we use equation (3) along with the contribution from the 1DES and contributions forε₋andε₊at the bias voltages at which these features are observed in the tunnelling conductance.

The 2DHF quantization was observed on the surfaces of different URu₂Si₂samples. In Extended Data Fig.5a–c, we show the result on another sample. Notice here that terraces have different sizes. We show in Extended Data Fig.5athe STM topography image. In Extended Data Fig.5b, we show a height profile through the white line in Extended Data Fig.5a。In Extended Data Fig.5c, we represent the tunnelling conductance along the central terrace (L ≈ 57 nm) of this profile. We observe similar tunnelling conductance curves as those presented in the main text. Notice the features atε₋andε₊。The quantized levels are also readily observed. These occur, however, at different energy values, as the size of the terraceLis different to that of the terrace in the main text. In Extended Data Fig.5d, we represent the values of the quantized levels found in terraces of different sizesLby different colours; we show the dispersion relation of the 2DHF as a magenta line.

In Extended Data Fig.5e, we show as coloured points the bias voltage dependence of the energy spacing ΔE连续量子化的l之间evels for terracesL₃,L₄, the terrace with lengthL = 57 nm (shown in Extended Data Fig.5c) and a terrace with lengthL = 27 nm (not shown). We can write that\(\Delta E={E}_{n+1}-{E}_{n}=\left(\frac{{\hbar }^{2}{\pi }^{2}}{2{m}^{* }{L}^{2}}\right)\left({(n+1)}^{2}-{n}^{2}\right)\), withn = 1, 2, 3,…. This gives a square-root dependence of ΔEon the energy,\(\Delta E={E}_{n+1}-{E}_{n}=\left(\frac{{\hbar }^{2}{\pi }^{2}}{2{m}^{* }{L}^{2}}\right)\left(2n+1\right)\propto \sqrt{E}\), shown in Extended Data Fig.5e。In Extended Data Fig.5f, we plot the average value of\(\frac{\Delta E}{2n+1}\)for each terrace as a function ofL。We find the expected\(\frac{1}{{L}^{2}}\)dependence.

Note that in Extended Data Fig.5and Fig.1d, we do not perform any symmetrization. We can see a tendency of the quantized states to shift towards the sides of the terrace, giving intensity patterns that are slightly asymmetric. We have calculated the expected patterns for different reflection coefficients at each side of the terrace. This produces asymmetric patterns similar to those observed experimentally. However, it is difficult to separate such an asymmetry from the signal coming from the edge states in the tunnelling conductance. Lateral symmetrization thus remains the best way to analyse and understand quantum states in the terraces observed here in URu₂Si₂。

We use\(\hbar /\tau ={\Gamma }_{0}+\left(| {E}_{0}| /4\pi \right){\left[(E+| {E}_{0}| )/{E}_{0}\right]}^{2}\)to fit the energy dependence of the lifetime⁶¹。The parameter |E₀|/4π is a prefactor that fits our experiment well. The prefactor is sometimes provided as a number²⁸and has also been estimated as\(\frac{{e}^{2}{k}_{{\rm{F}}}^{2}\pi }{4\pi {{\epsilon }}_{0}\,32\,\hbar {q}_{{\rm{TF}}}}\)or, equivalently,\(\frac{e{m}^{3/2}}{32\times {3}^{5/6}{\pi }^{2/3}{{\epsilon }}_{0}^{1/2}{\hbar }^{4}{n}^{5/6}}\)(withϵ₀the dielectric constant,nthe electron density andq_TFthe Thomas–Fermi screening length)²⁷。These estimations provide similar values to |E₀|/4π.

To obtain the energy dependence of the reflection coefficient,r(E), we used the model described in ref. ²⁴。To this end, we consider a one-dimensional periodic array of scattering objects, each modelled by a square potential well of widthb < L(Lis the width of the terrace). A constant complex potentialWprovides confinement and coupling to the bulk states. We can then write

with\(k=\sqrt{\frac{2{m}^{* }E}{{\hbar }^{2}}}\)and\(q=\sqrt{\frac{2{m}^{* }(E-W)}{{\hbar }^{2}}}\)。The reflection coefficientr(E) is given byr(E) = |R(E)|²。For the dashed line in Fig.2e, we use typical parameters for Cu, withm* = 0.46m₀andW = (−2 − 1i) eV. We shift the obtained curve in energy to obtain a result within the energy range of our data. For the continuous line in Fig.2e, we usem* = 17m₀andW = (−18 − 5i) meV.

Band-structure calculations at U-terminated surfaces of URu₂Si₂

The band structure of bulk URu₂Si₂has been analysed previously in detail using DFT calculations^62,63,64。Relevant results coincide with angle-resolved photoemission, STM and quantum oscillation studies^{22,65,66,67,68,69,70,71}。

Several surface states have been observed by angle-resolved photoemission spectroscopy^19,20,21,22。The surface state discussed in refs. ^19,20is formed by a hole-like band with its maximum at −35 meV and is thus far from what we observe here. At theXpoint of the Brillouin zone, there are no bulk states. Angle-resolved photoemission spectroscopy measurements show hints of surface-like bands with two-dimensional character at these points²¹。We have taken a closer look at theXpoint through DFT calculations. To this end, we built a U-terminated supercell consisting of 37 atomic layers, giving a total of ten U layers (Extended Data Fig.6a）.We performed DFT calculations using the full-potential linearized augmented plane-wave method with local orbitals as implemented in the WIEN2k package⁷²。Atomic spheres radii were set to 2.5, 2.5 and 1.9 Bohr radii for U, Ru and Si, respectively. We used a 19 × 19 × 1 mesh ofk-points in the first Brillouin zone, reduced by symmetry to 55 distinctk-points. The RK_maxparameter was set to 6.5, resulting in a basis size of approximately 5,400 (more than 100 basis functions per atom). Spin–orbital coupling was included in the second variational step⁷³和当地轨道包括了相对论U 6p_1/2and Ru 4p_1/2states. The basis for calculations of the spin–orbital eigenvalue problem consisted of scalar-relativistic valence states of energies up to about 5 Ry, resulting in a basis size of about 3,800. The local density approximation was used for the treatment of exchange and correlation effects^63,74。

In Extended Data Fig.6b, we highlight in particular the U spin-up character of the obtained surface-projected band structure. The spin-down character is much less pronounced within the shown energy range. There are several bands inside gaps of the bulk band structure, but only those around theXpoint of the simple tetragonal Brillouin zone,X_st(see Extended Data Fig.6c), are sufficiently shallow to provide large effective masses.

We find a surface state (upper inset of Extended Data Fig.6b),包括两个杂交孔乐队,形式ing an M-shaped feature close to the Fermi level. The dispersion relation found in our experiment (magenta line in the upper inset of Extended Data Fig.6b) is compatible with the central part of the M-shaped feature.

1DES and HO within U layers

To analyse the 1DES at the step between two terraces, we use a one-dimensional Dirac-function-like potential at the step,V(x) = U₀δ(x − x_1DES), in whichx_1DESis the position of the 1DES. We takeU₀ = b₀V₀, withb₀the width of the potential well andV₀the energy depth (V₀ < 0). We add a complex potential,V(x)→(U₀ − iU₁)δ(x − x_1DES) to simulate the coupling of the 1DES to the bulk of the crystal. A schematic representation of this model is shown in Extended Data Fig.7a。Solving the Schrödinger equation forE < 0, we obtain the Green’s function of the states in the potential well

in which\({\lambda }_{x}=\frac{{\hbar }^{2}}{{m}^{* }}| {U}_{0}{| }^{-1}\)is the decay length withm* the effective mass.E_1DESandη_1DESare the energy position and the energy broadening of the 1DES given by

in whichδVis the height of the potential barrier of the well relative to the Fermi level.

We can now fit the tunnelling conductance at the 1DES using

convoluted with the derivative of the Fermi–Dirac distribution function. Extended Data Table1shows the extracted fitting parametersE_1DES,η_1DES,λ_xandx_1DESfor the four different terracesL₁toL₄from Fig.1。

From Extended Data Table1, we see that the energy position and the energy broadening of the 1DES are independent of the terrace size, with average values ofE_1DES = −0.52 ± 0.14 meV andη_1DES = 0.45 ± 0.06 meV. We also see that all the spatial features are always at the same position with respect to the step,x_1DES ≈ 4.0a₀,a₀being the in-plane lattice constant, with a decay lengthλ_x ≈ 0.9 nm ≈ 2a₀。The latter indicates that 1DESs and 2DHFs couple when the decay length reaches a few interatomic distances. With the extracted average values from Extended Data Table1forλ_x,η_1DESandE_1DES, we obtainU₀ = 5.4 meVÅ,U₁ = 0.38 meVÅ andδV = 3.1 meV.

We can analyse the 1DES through the tunnelling conductance at a step (Extended Data Fig.7b,c）.At low bias voltages, we find a dip in the tunnelling conductance of a few nanometres at the upper side of the step (blue lines in Extended Data Fig.7b; for example, at −1.2 mV). The dip fills with the 1DES at aboutE_1DES(red lines in Extended Data Fig.7bat −0.4 mV) and empties again at higher bias voltages. This shows that charge depletion close to the step opens a gap in the band structure. The gap is filled at the resonant energy of the 1DES, as observed previously in metals^30,75,76。By normalizing the tunnelling conductance to its shape far from the step (Extended Data Fig.7c), we can follow the decay of the 1DES into the quantum-well states of the 2DHF with the model described above (Extended Data Fig.7a）.The decay length is on the order of the inverse of the wavevector of the 2DHF.

Taking a closer look at the steps, we surprisingly find a notable in-plane anisotropy of the 1DES. As we see in Extended Data Fig.7b, the 1DES is observed when crossing steps along the dashed red line in Extended Data Fig.7bbut not along the dashed blue line, as discussed in the main text. It is useful here to take a closer look at the HO as well. As discussed in refs. ^{14,34,64,77,78,79}, there is no dipolar (magnetic) or structural order related to the HO phase. Instead, the U lattice can present some sort of long-range electronic ordering, whose actual symmetry and shape is considered as a relevant and open mystery¹⁴。

Previous NMR measurements indicated the absence of fourfold in-plane symmetry breaking in bulk URu₂Si₂(ref. ³⁵), whereas our STM data clearly show an in-plane symmetry breaking in the 1DES. At the surface, there can be marked changes in the electronic structure owing to a modification of the valence of uranium atoms^21,80。Rather, the breaking of the in-plane symmetry observed here in the 1DES suggests that a fundamental breaking of the near-surface electronic properties of the U lattice is at play in the HO phase.

Interplay between superconductivity and the 2DHF

We consider several parallel conduction channels between the tip and the surface. For simplicity, we take into account tunnelling into the 2DHF and into the feature of largest size atε₋(Extended Data Fig.8a）.The first channel,t₁, connects the tip with the 2DHF. The 2DHF is superconducting by proximity from the bulk superconductor, which we model using a couplingt_s。With the second channel,t₂, we connect the tip to other surface features. We can write the retarded Green function\({\hat{G}}^{{\rm{r}}}\)as

with

in whichE_2DHFis the energy associated to the 2DHF and includes the shift of energy owing to HO and Fano resonance,Wis an energy scale related to the normal density of states at the Fermi level byρ(E_F) = 1/(πW), Δ is the superconducting gap andηis a small energy relaxation rate. We have added the self energiesiΓ_j/2, (j = 2DHF, −, +), with Γ_jthe width of the resonancej。

The differential conductance is calculated as

with

Heref(E, T) is the Fermi–Dirac distribution at the energyEand temperatureTand\({\sigma }_{0}=\frac{2{e}^{2}}{h}\)is the quantum of conductance (withhbeing Planck’s constant andethe elementary charge). Notice thatTis the transmission, not the density of states often used to discuss STM measurements in superconductors. Notice also that we take into account tunnelling into the 2DHF (\({G}_{11}^{{\rm{r}}}\)) and intoε₋(\({G}_{33}^{{\rm{r}}}\)), with mixed contributions (\({G}_{31}^{{\rm{r}}}\)and\({G}_{13}^{{\rm{r}}}\)）.

To fit the tunnelling conductance curves shown in Fig.4a,band Extended Data Fig.8b, we subtracted an asymmetric background (see Extended Data Figs.2and8b）.In Extended Data Table2, we show the parameters used to obtain the tunnelling conductance with temperature (shown as black lines in Fig.4a,b) from equation (11）.We do not vary the parametersη = 0.018 meV,ε_2DHF = 12 meV, Γ_2DHF = 1 meV, Γ₋ = 0.55 meV and Γ₊ = 0.14 meV with temperature. We see that the superconducting lifetime itself is practically negligible,η = 0.018 meV ≪  Δ = 0.2 meV. The large zero-bias conductance is not because of the incomplete coupling to the bulk, ast_sis close to one. There is further smearing coming from features atε₊andε₋。Γ₊and Γ₋provide the smeared superconducting density of states and a finite tunnelling conductance at zero bias. Notice that the superconducting gap vanishes at the critical temperatureT_cbut that the strongly bias-voltage-dependent tunnelling conductance remains up to higher temperatures (Extended Data Fig.8）.

There are numerous evidences for d-wave or more complex superconducting properties in URu₂Si₂。超导密度的差异f states between these superconducting states and s-wave superconductivity are relatively small, particularly because the tunnelling conductance obtained with the model described here provides smeared conductance curves. The same occurs for the temperature dependence of the superconducting gap. Instead, the shape and asymmetry of the tunnelling conductance at defects might provide the connection between the properties of the superconducting 2DHF and the unconventional superconductivity of the bulk.

Results at point defects

We analyse in more detail here the tunnelling conductance at defects. We plot the tunnelling conductance obtained on two different defects in Extended Data Fig.9aas blue and red lines. Notice the pronounced electron–hole asymmetry, which provides curves that widely differ from the curves far from defects (black line in Extended Data Fig.9a）.As discussed above, we can identify two kinds of defects: protrusions with height increases of around 15 pm, probably because of interstitial atoms located beneath the surface (Extended Data Fig.9b,d,f,h的下午15点左右),波谷大小,可能because of vacancies beneath the surface (Extended Data Fig.9c,e,g,i）.The defects visibly affect the tunnelling conductance. We plot the tunnelling conductance atε₊, 0 mV andε₋for both types of defect in Extended Data Fig.9f,g。In Extended Data Fig.9h,i, we show the spatial dependence of the tunnelling conductance along a crystalline axis for both types of defect. At the site of the defect, there is a pronounced electron–hole asymmetry, which is opposite for each kind of defect. Protrusions provide a substantially enhanced tunnelling conductance for empty states above the Fermi level, whereas troughs provide the opposite (Extended Data Fig.9a）.At zero bias, the troughs show a pronounced in-plane anisotropy and a reduction of the superconducting gap, whereas the protrusions are in-plane isotropic and show an opened superconducting gap. When leaving the defect, the usual behaviour is recovered after several nanometres (Extended Data Fig.9h,i）.

To explain the pronounced modification of the electron–hole asymmetry of the tunnelling conductance, let us consider electron correlations creating a large Fermi surface scenario at some portion of the band structure. At low temperatures, correlations provide an avoided band crossing owing to hybridized heavy and light bands. We can assume that it happens somewhere in the band structure of URu₂Si₂, for example, close to the Γ point, as suggested in refs. ^14,18,47。Then, we obtain at low temperatures a large Fermi surface with heavy electrons and a close-lying light band with smaller wavevectors above the Fermi level. We can then assume that both kinds of defect couple to different parts of such a correlated band structure. Following our experiments, protrusions create 2DHF coupling to the small light band and a large density of states for empty states above the Fermi level (red curve in Extended Data Fig.9a）.Troughs can instead favour coupling to the heavy band, giving the opposite behaviour (blue curve in Extended Data Fig.9a）.The superconducting gap is disturbed at troughs. Generally, we expect all sorts of defects to be pair breaking, as URu₂Si₂is not a s-wave superconductor. However, two-dimensional quantized states screen pair-breaking interactions from the underlying superconducting bulk⁸¹。This suggests that the absence of in-gap states in protrusions is because of screening by the 2DHF. Troughs, however, produce a strong coupling to the heavy bands that carry the heavy superconducting state and thus also contribute to pair breaking.

In-gap states at troughs have a certain in-plane anisotropy (Extended Data Fig.9g, middle panel). The anisotropy of the superconducting gap has been analysed with macroscopic measurements^82,83,84,85。There are indications for gap nodes, for instance, from specific heat measurements^82,83,85。Local vortex dynamics shows an in-plane fourfold or twofold pinning potential⁸⁶。When measured as a function of the angle, there is a pronounced out-of-plane anisotropy, which has been associated to nodes along thecaxis, but there is little in-plane variation⁸⁵。Following such a nodal structure, several experiments propose a chiralk_z(k_x + ik_y) superconducting wavefunction^84,85,87。The surface states of a (k_x ± ik_y) superconductor are predicted to be chiral arc states connecting the Weyl nodes⁸⁸。,目前还不清楚这些表面的状态the superconducting phase adapt to the surface states of the normal phase, which appear as a consequence of the surface-induced perturbation of the crystalline potential. As mentioned, we observe an asymmetry at zero bias (Extended Data Fig.9g, middle panel). To increase the signal-to-noise ratio, we were forced to apply a fourfold symmetrization. Thus, the anisotropy might be twofold or fourfold. The chiralk_z(k_x + ik_y) state is in-plane isotropic. However, the shape of in-gap states is determined by the anisotropy of the normal-state wavefunctions, together with the symmetry of the superconducting wavefunction, so that the observed in-plane anisotropy of the in-gap states probably reflects the normal-state in-plane anisotropy.